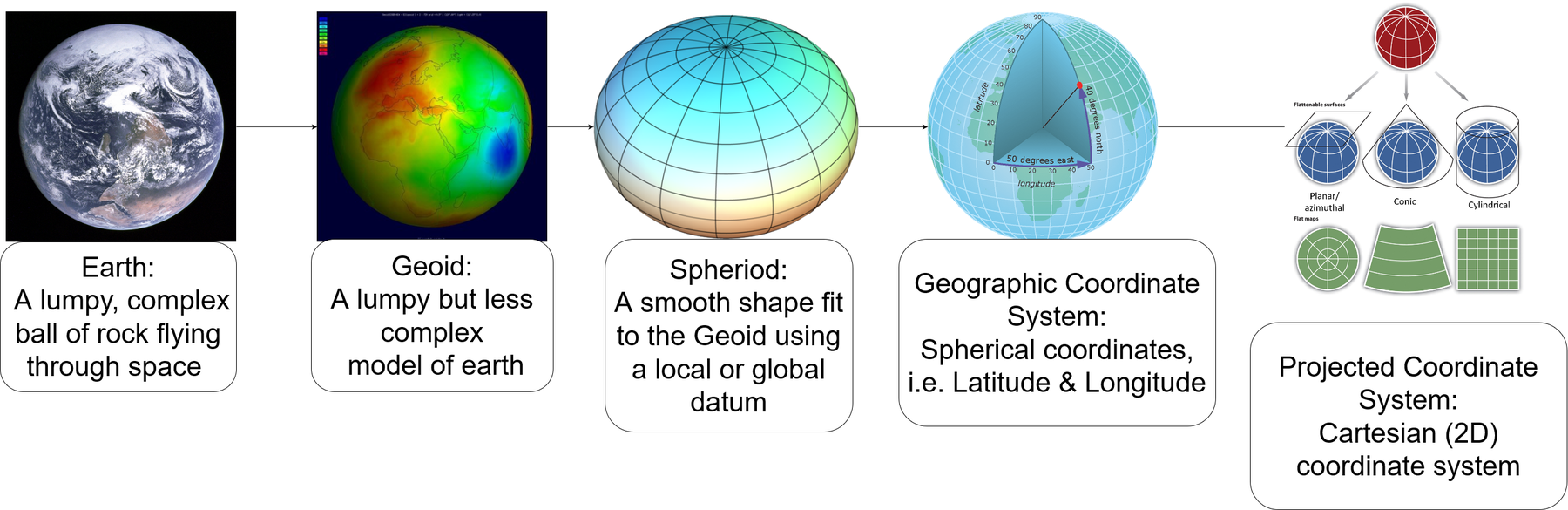
Steps of Abstraction
From the 3D Earth to 2D maps.
Flattening the Blue Marble
This is what earth looks like when you take a picture of it from 29,000 kilometers away.
- This photo is called the Blue Marble, it was taken from Apollo 17

Flattening the Blue Marble
This is the version that was published.
- NASA rotated the image 180 degrees to fit peoples expectations

Complexities of Earth
A lumpy ball of rock.
- The earth isn’t “round”!
- It has multiple distortions caused by:
- Topography
- Gravity Differentials
- Centrifugal Force
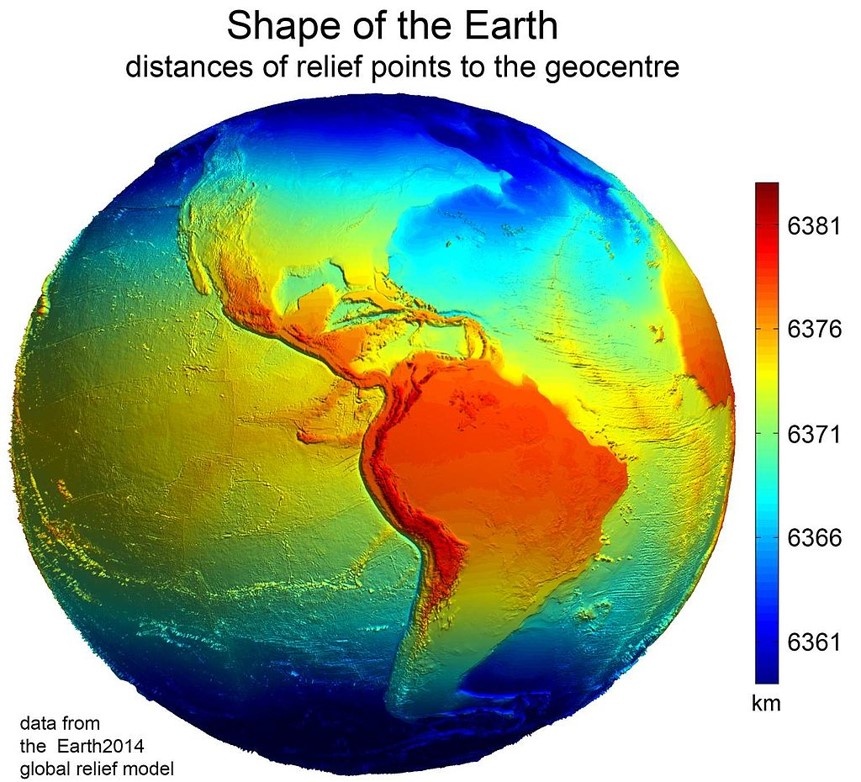
Our Infinitely Complex Earth
Cartographers have developed strategies for dealing with these distortions:
- Topography
- Gravity Differentials
- Centrifugal Force

Ignore Topography!?
Topography is very localized, in most applications it isn’t explicitly needed to make a map.
- We can account after the fact if needed
- Digital Elevation Models
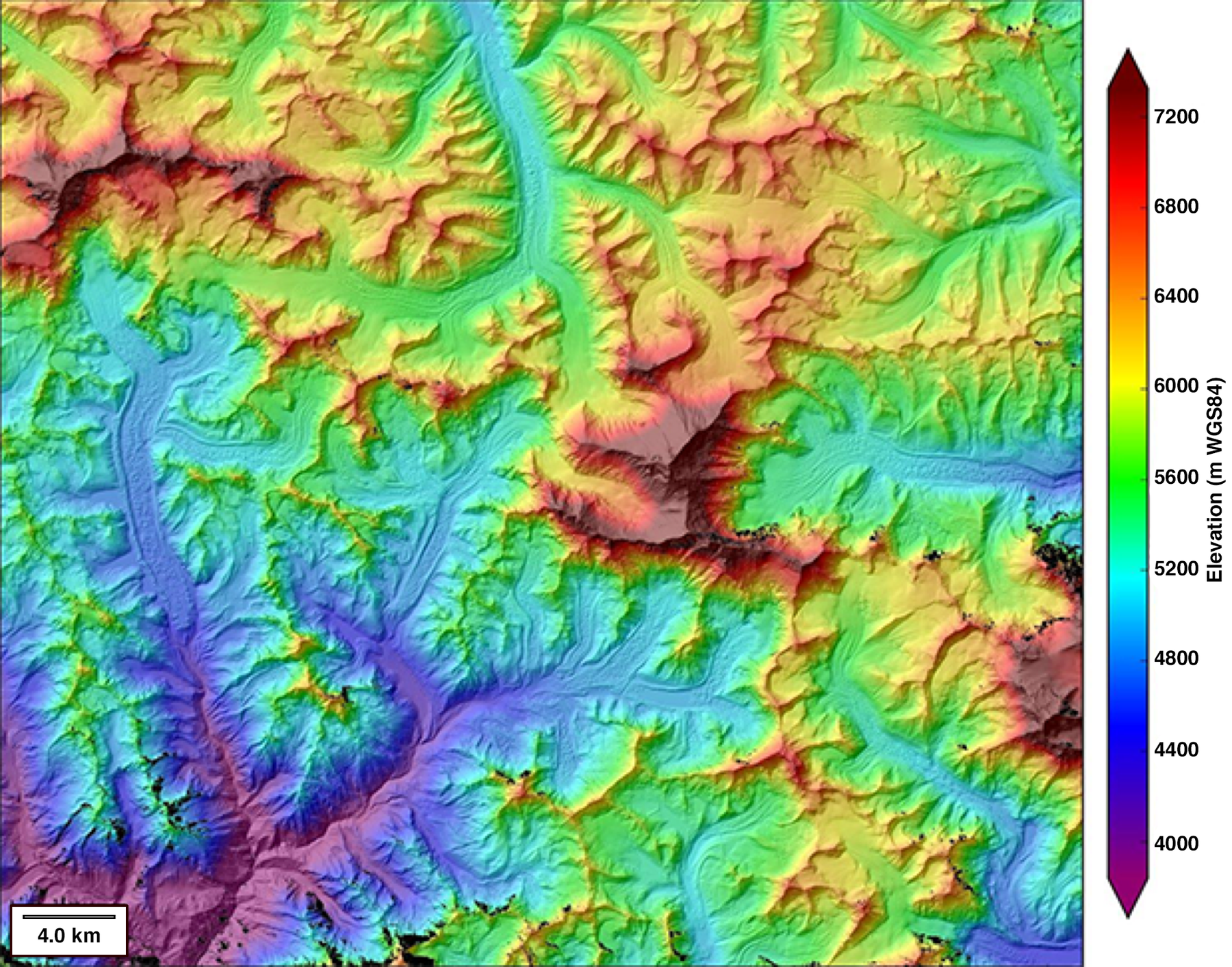
DEM of Mt. Everest
Ignore Topography!?
Topography is very localized, in most applications it isn’t explicitly needed to make a map.
- We can account after the fact if needed
- Digital Elevation Models
- Contour Lines
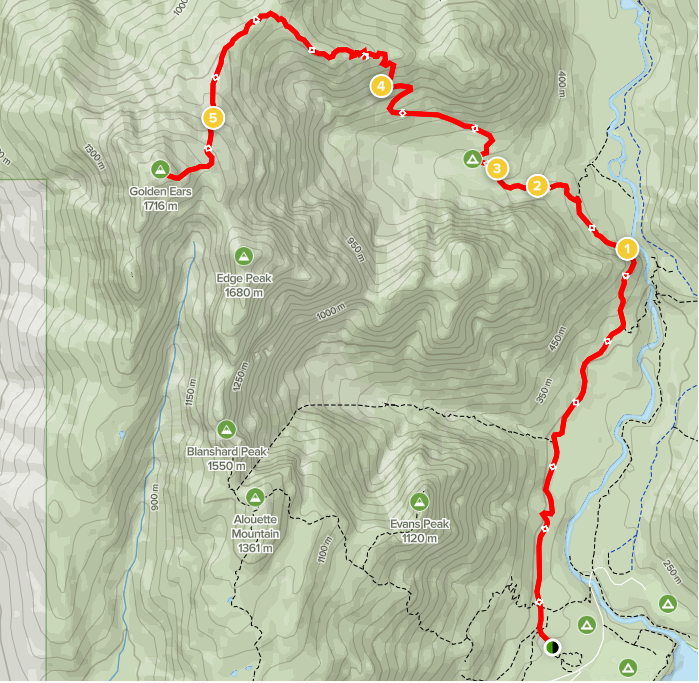
Golden Ears Trail
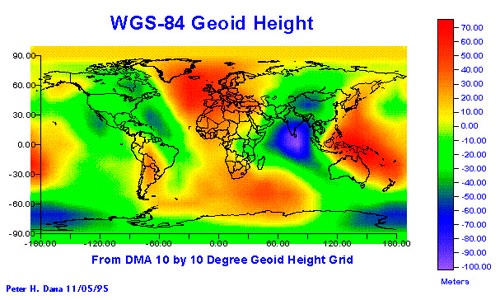
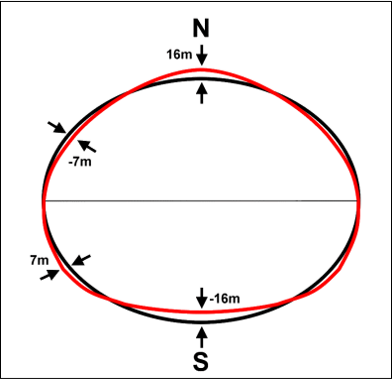
The Geoid
A simplified model that ignores due to topography.
- Gravity differentials: Earth’s crust is not uniformly dense
- Surface to “sinks” down or “floats” up
- Continental scale:
- +85 m to −106 m
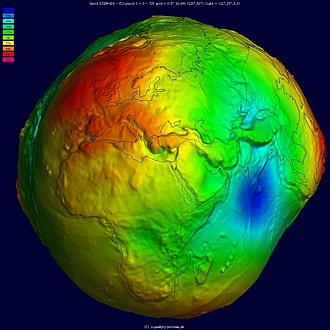
Vertical scale exaggerated to show gravity induced elevation differences.
The Geoid
A simplified model that ignores due to topography.
- Gravity differences measured by satellites
![]()
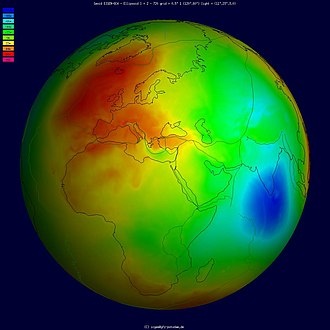
The Geoid without vertical exaggeration.
TopHat Question 1
The Geoid accounts for elevation differences in the Earth’s crust due to
- Topography
- Centrifugal force
- Density differences
- All of the above
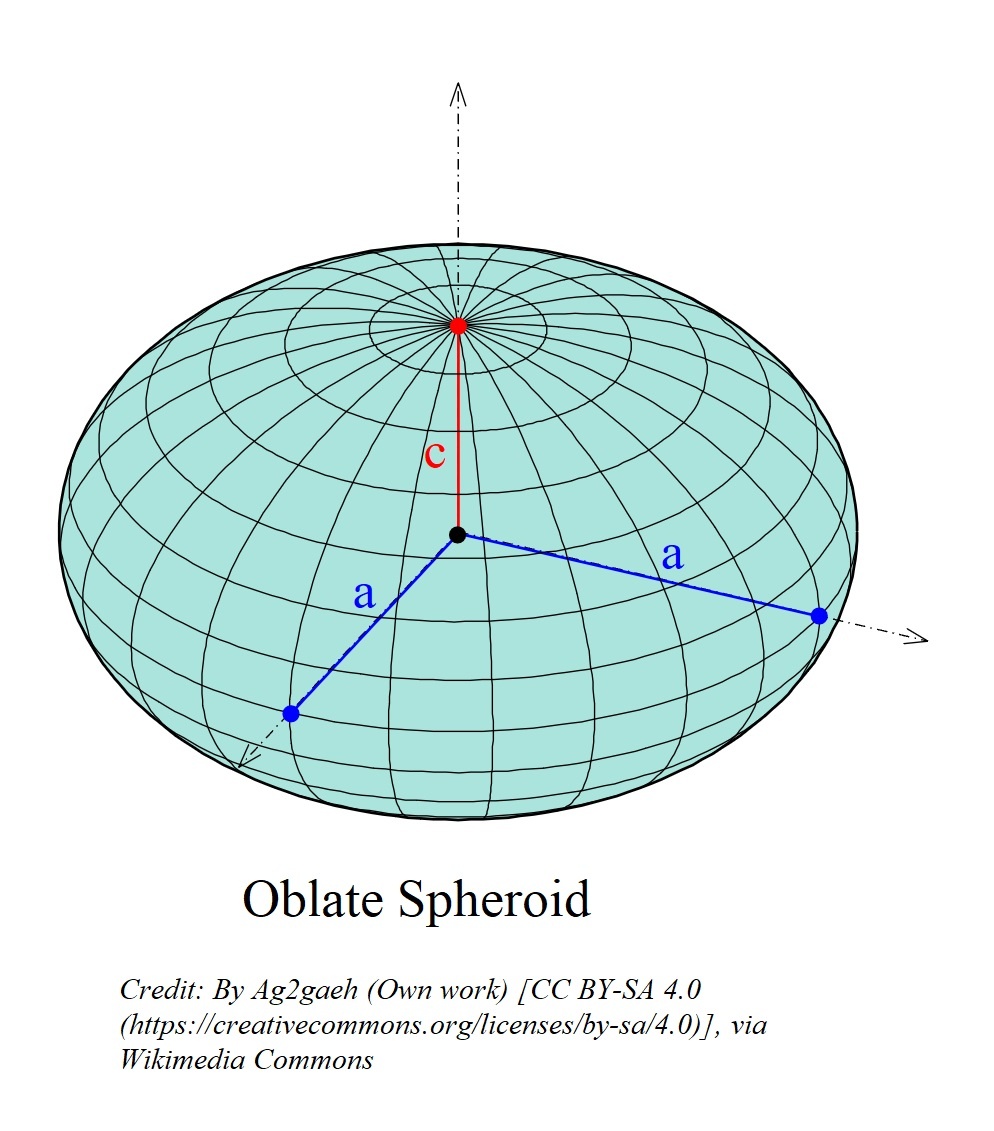
The Oblate Spheroid
Due to Centrifugal Force the earth is ~ 26 km wider at the equator
- A close approximation of Earth’s real shape
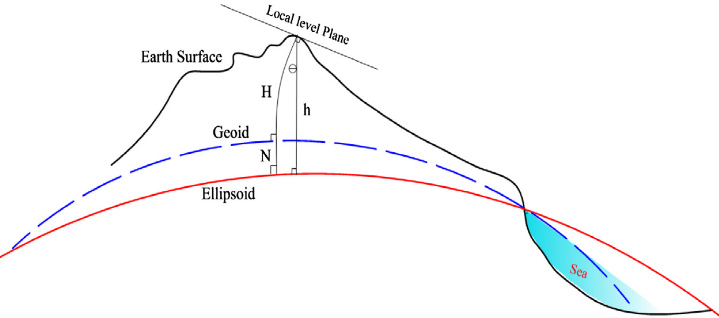
Datums
Measuring distance/height requires a reference point.
- A Datum is the reference system
- Gives meaning to coordinates
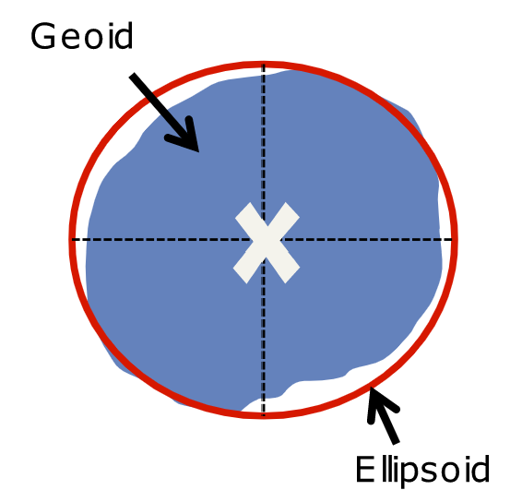
Datums
Fit a spheroid to the geoid using a Datum.
Explicitly account for effects of Centrifugal force
Minimize distortion from density differentials
“Ignore” topography.
- Can be Global or Local
Reference point to account for distortion
Global Datums
The center of the geoid is the reference point.
- Fits fairly well everywhere
![]()
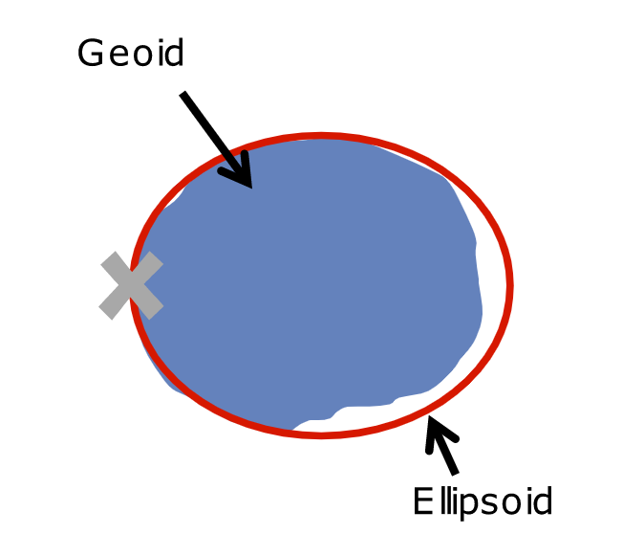
Local Datums
A location on the geoid’s surface the reference point.
- Fits very well in one region
Why Does it Matter?
Generally speaking:
- Global maps always use a global datum
- Local datums are better for specific regions
- Global datums can work
- But less accurate

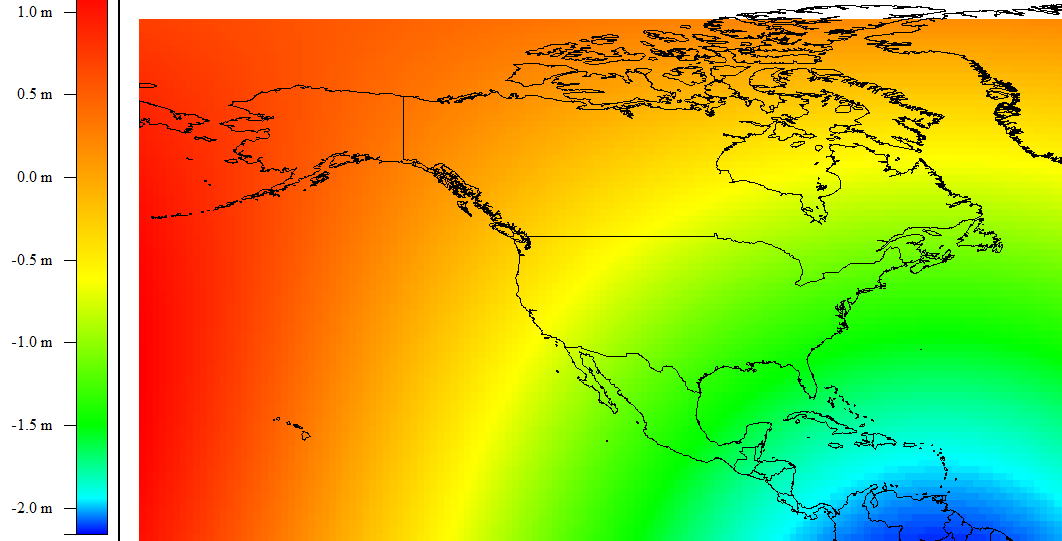
Why Does it Matter?
Only minor differences between in North America, larger differences elsewhere.

TopHat Question 2
A _____ datum is fixed to the center of the geoid while a _____ datum is fixed to a point on the geoid’s surface.
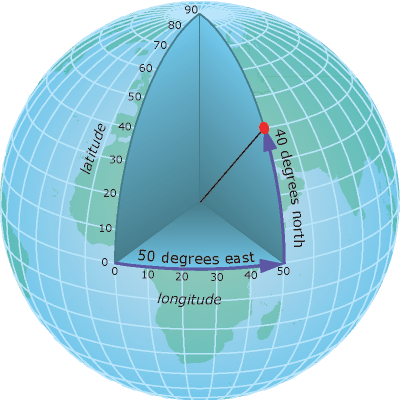
Geographic Coordinate System
Latitude/Longitude is a Geographic Coordinate System (GCS).
- Location on the surface of a 3D object with only 2 values
- Fixed to the surface of spheroid
- Not the actual earth’s surface
Latitude
Angular distance from the equator
- -90°(South) to +90°(North)
- Often called parallels

Longitude
Angular distance from the prime meridian
- -180° (West); to +180° (East)
- Often called meridians

Latitude & Longitude
Sometimes refereed to as a graticule.
Degrees Minutes Seconds
- Vancouver BC:
- 49°15′40″N 123°06′50″W
- Sydney NSW:
- 33°51′54″S 151°12′34″E
Decimal Degrees
- Vancouver BC:
- 49.261, -123.113
- Sydney NSW:
- -33.865, 151.209
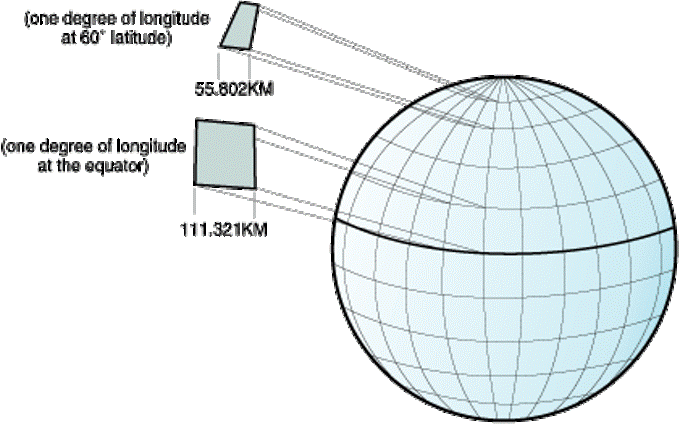
Meridians converge at the poles!
Distance between degrees of longitude decreases with increasing latitude.
- You can’t accurately display a Geographic Coordinate System on a 2D surface (map/screen).
- This is why we need map projects!
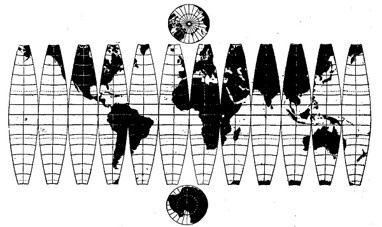
Making a Flat Map
Displaying Lat/Lon in 2D doesn’t work, things to look “scrunched”
We have to Project our map.
- Intentionally distort data to display in 2D
TopHat Question 3
Lines of latitude converge at the poles.
- True
- False
Projected Coordinate Systems
A map projection is a mathematical transformation used to “flatten” a geographic coordinate system.
- Imagine sending rays of light through the ellipsoid onto a flat surface, the resulting image is a projection

Making a Flat Map
Applying a projection:
- Converts to linear units
- Allows distance/area calculations
- Makes things look better
TopHat Question 4
A Geographic Coordinate System is a mathematical transformation we apply to project the earth on a 2D plane.
- True
- False
Steps of Abstraction